Grade 12 | Lesson 14

Mathematics

Lesson Overview

Pre-Calculus

• Graphs of the Trigonometric Functions

Pre-Calculus

Graphs of the Trigonometric Functions
We live in an electrical age. Electricity powers our lights, heats our homes, and even runs our cars. However, unlike light or sound, under normal circumstances we cannot sense this form of energy directly. We must use tools, like an oscilloscope, to measure the behavior of electric currents. The electric power that is used in American homes is delivered in alternating current. An alternating current surges in one direction and then the other in a way that can be represented on the oscilloscope by a sine curve.

You could generate the coordinates of points on the parent graphs of trigonometric functions with a calculator or spreadsheet program. The graphs may be generated by using a graphing calculator or graphing software. The parent graphs of the six basic trigonometric functions are shown below.

Do you notice a similarity between the sine and cosine curves? The cosine curve is the sine curve translated 90° to the left along the x axis. Since the cosecant and secant ratios are the reciprocals of the sine and cosine ratios, the same relationship holds true for their curves. However, this does not hold true for the tangent and cotangent curves, why?

Quadrantal Angles
A quadrantal angle is an angle in standard position whose terminal side coincides with one of the coordinate axes. So the measures of the quadrantal angles are: -270°, -180°, -90°, 0°, 90°, 180°, 270°, etc... Knowing the characteristics of the basic trigonometric graphs can help you quickly determine quadrantal values of the trigonometric functions.

The value of the sine function is 0 at -360°, -180°, 0°, 180°, 360°, and at all other integral multiples of 180°. The maximum value of the sine function is 1 for 90° or -270°, and the minimum is -1 for 270° or -90°. The fact that the trigonometric functions are periodic allows us to find more function values easily.

Periodic Function and Period
A function is periodic if, for some real number a: f(x + a) = f(x) for each x in the domain of f.

The least positive value of a for which f(x) = f(x + a) is the period of the function.

Since the sine function is periodic, its graph repeats itself every 360°. So we can find other zero, maximum, and minimum values easily. For example, since we know that sin 90° = 1, sin(90° + 360k°) = 1 for any integral value of k. The same methods can be used to find special values of the other functions.

Here is an example:

Let's use the graph of the cosine to find the values of q for which cos q = 1.

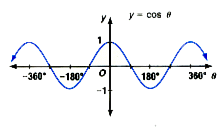
When cos q = 1 and -360 < q < 360°, the value of q is -360°, 0°, or 360°. Since the cosine function has a period of 360°, the values of q for which cos q = 1 are given by 0° + 360k° or simply 360k°, where k is any integer.

The graph of a trigonometric function may be drawn from the knowledge of its shape and the values of the function at integral multiples of 90°.